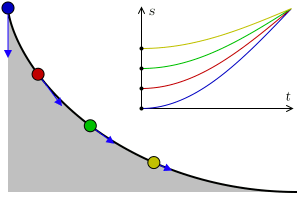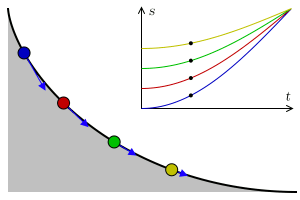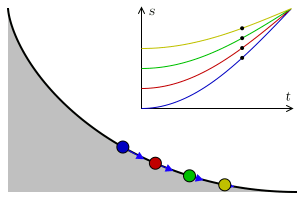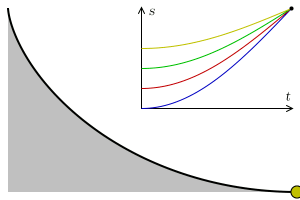
Fájl:Tautochrone curve.gif
Tautochrone_curve.gif (300 × 200 képpont, fájlméret: 102 KB, MIME-típus: image/gif, ismétlődik, 80 képkocka, 3,2 s)
Fájltörténet
Kattints egy időpontra, hogy a fájl akkori állapotát láthasd.
| Dátum/idő | Bélyegkép | Felbontás | Feltöltő | Megjegyzés | |
|---|---|---|---|---|---|
| aktuális | 2009. augusztus 1., 14:15 | 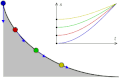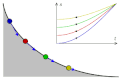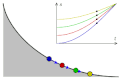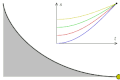 | 300 × 200 (102 KB) | Geek3 | new physically correct version |
| 2007. május 9., 07:56 | 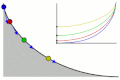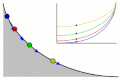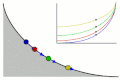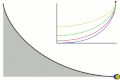 | 300 × 200 (602 KB) | Rocchini | {{Information |Description=Tautochrone curve animation (4 points runs over a cycloid) |Source=Own work |Date=2007-05-09 |Author=Claudio Rocchini |Permission=CC-BY 2.5 }} |
Fájlhasználat
Az alábbi lap használja ezt a fájlt:
Globális fájlhasználat
A következő wikik használják ezt a fájlt:
- Használata itt: ar.wiki.x.io
- Használata itt: bn.wiki.x.io
- Használata itt: ca.wiki.x.io
- Használata itt: da.wiki.x.io
- Használata itt: de.wiki.x.io
- Használata itt: de.wikiversity.org
- Használata itt: en.wiki.x.io
- Használata itt: es.wiki.x.io
- Használata itt: fa.wiki.x.io
- Használata itt: fr.wiki.x.io
- Használata itt: fr.wikibooks.org
- Használata itt: fr.wiktionary.org
- Használata itt: he.wiki.x.io
- Használata itt: hr.wiki.x.io
- Használata itt: id.wiki.x.io
- Használata itt: it.wiki.x.io
- Használata itt: ja.wiki.x.io
- Használata itt: kk.wiki.x.io
- Használata itt: ko.wiki.x.io
- Használata itt: lt.wiki.x.io
- Használata itt: lv.wiki.x.io
- Használata itt: nl.wiki.x.io
- Használata itt: nn.wiki.x.io
- Használata itt: no.wiki.x.io
- Használata itt: pl.wiki.x.io
- Használata itt: pt.wiki.x.io
- Használata itt: ro.wiki.x.io
- Használata itt: ru.wiki.x.io
- Használata itt: sl.wiki.x.io
- Használata itt: tr.wiki.x.io
- Használata itt: www.wikidata.org
- Használata itt: zh.wiki.x.io