Wikipédia

Fájl:Znam-2-3-11-23-31.svg

Eredeti fájl (SVG fájl, névlegesen 396 × 396 képpont, fájlméret: 7 KB)

Ez a fájl a Wikimedia Commonsból származik. Az alább látható leírás az ottani dokumentációjának másolata.

A Commons projekt szabad licencű kép- és multimédiatár. Segíts te is az építésében!

Összefoglaló

Leírás Graphical demonstration that 1 = 1/2 + 1/3 + 1/11 + 1/23 + 1/31 + 1/(2×3×11×23×31). Each row of squares has k squares of side length 1/k, for some k in the set {2,3,11,23,31,47058}; for instance the first row has two squares of side length 1/2. Thus, each row of squares has area 1/k, and all six rows together exactly cover a unit square. The bottom row, with 47058 squares of side length 1/47058, would be too small to see in the figure, and is not shown. Sets of integers such that , such as the set {2,3,11,23,31} used to construct this figure, correspond to solutions of Znám's problem. As all numbers in the set {2,3,11,23,31} are prime, their product 47058 is a primary pseudoperfect number.
Dátum 2006. december 5. (eredeti feltöltésének dátuma)
Forrás Áthozva az en.wikipedia projektből a Commonsba.
Szerző David Eppstein a(z) angol Wikipédia projektből
Más változatok File:Sylvester-square.svg

Licenc

Public domain David Eppstein a(z) angol Wikipédia projektből, a mű szerzője művét közkinccsé nyilvánította. Ez a világ minden részén érvényes.
Egyes országokban ez jogilag nem lehetséges. Ha így van, akkor:
David Eppstein jogot ad bárkinek, hogy bármilyen célból, feltétel nélkül használhassa ezt a fájlt, kivéve a törvény által kötelezően előírt feltételeket.

Eredeti feltöltési napló

Az eredeti leírólap itt volt. Az itt következő felhasználónevek az en.wikipedia projektre hivatkoznak.
  • 2006-12-05 01:51 David Eppstein 256×256×0 (5661 bytes) Graphical demonstration that 1 = 1/2 + 1/3 + 1/11 + 1/23 + 1/31 + 1/(2×3×11×23×31). Each row of squares has k squares of side length 1/k, for some k in the set {2,3,11,23,31,47058}; for instance the first row has two squares of sid

Képaláírások

Adj meg egy egysoros magyarázatot arról, hogy mit mutat be ez a fájl

A fájl által ábrázolt elemek

mű tárgya

létrejöttének ideje

5. december 2006

Fájltörténet

Kattints egy időpontra, hogy a fájl akkori állapotát láthasd.

Dátum/időBélyegképFelbontásFeltöltőMegjegyzés
aktuális2007. augusztus 25., 03:27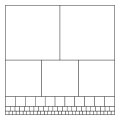Bélyegkép a 2007. augusztus 25., 03:27-kori változatról396 × 396 (7 KB)David Eppstein{{Information |Description=Graphical demonstration that 1 = 1/2 + 1/3 + 1/11 + 1/23 + 1/31 + 1/(2×3×11×23×31). Each row of squares has k squares of side length 1/k, for some k in the set {2,3,11,23,31,47058}; for instance the first row has two squares

Az alábbi lap használja ezt a fájlt:

Globális fájlhasználat

A következő wikik használják ezt a fájlt:

A lap eredeti címe: „https://hu.wiki.x.io/wiki/Fájl:Znam-2-3-11-23-31.svg”